Indexing Cam Angles Comparison (2/2)

In post [Indexing Cam Angles Comparison (1/2)], we explained how to calculate the displacement of indexer (h_m) from number of stops (S) and the meaning of the indexing angle (b_m). In this post, we will show how the rotary indexers with different indexing angles move.

We have 2 indexers. Both of them have S = 4, but the first indexer has b_m = 120 deg. and the second indexer has b_m = 240 deg.

Indexers with b_m=120 deg. and b_m=240 deg.

The input shafts of both indexers rotate continuously at the same speed. The first indexer completes its indexing in 120 deg. while the second indexer takes longer time and completes its indexing in 240 deg. We can clearly see from the animation that the longer the indexing time, the smoother indexing motion (lower acceleration).

The indexer has many displacement profiles e.g. modified sine, modified trapezoidal, etc. But for the explanation, we will use cycloidal motion profile.

The cycloidal displacement profile can be expressed as ...

... (eq. 1)

where:
h = displacement (deg.)

q = angle of the input shaft (deg.)
h_m = displacement of the turret plate (deg.)
b_m = indexing angle (deg.)

Displacement diagram of 2 indexers with different indexing angles (b_m)

We can see from the displacement diagram that both curves have smooth continuous displacement. There is not much displacement at approximate 10% and 90% of the indexing angle.

• For b_m = 120 deg.: 10% = 12 deg.
• Very small displacement within first 12 deg. and from 108 deg. to 120 deg.
• For b_m = 240 deg.: 10% = 24 deg.
• Very small displacement within first 24 deg. and from 216 deg. to 240 deg.

This fact can be used for timing diagram design later.

Maximum velocity can be calculated from ...

... (eq. 2)

where:
v_max = maximum velocity (deg./s)
h_m = displacement of the turret plate (deg.)

w = angular velocity of the input shaft (rad/s)
t_m = indexing time (s) -- indexing time is proportional to indexing angle (b_m)

Velocity diagram of 2 indexers with different indexing angles (b_m)

Both indexers have same number of stops (same displacement h_m) and same input velocity (w). So the relationship between velocities can be expressed as

... (eq. 3)

Since the indexing time of the second indexer is 2 times the first one. The velocity of the second indexer then becomes half of the first one as can be seen in eq. 3

The maximum tangential acceleration of cycloidal profile when the input shaft rotates at a constant speed (constant w) can be expressed as ...

... (eq. 4)

where:
a_max = maximum tangential acceleration (deg./s²)
h_m = displacement of the turret plate (deg.)

w = angular velocity of the input shaft (rad/s)
t_m = indexing time (s) -- indexing time is proportional to indexing angle (b_m)

Both indexers have the same number of stops (same displacement h_m) and same input velocity (w). So the relation between accelerations can be expressed as

... (eq. 5)

Since the indexing time of the second indexer is 2 times the first one. The acceleration of the second indexer then becomes 1/4 of the first one as can be seen in eq. 5 -- This is quite interesting. By selecting longer indexing angle (indexing time), we can reduce its acceleration by square of indexing time ratio.

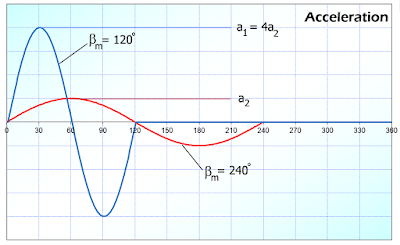Acceleration diagram of 2 indexers with different indexing angles (b_m)

In this example, the second indexer runs at only 25% tangential acceleration of the first indexer by selecting 2 times indexing angle. However, there will be less time during dwell period for other machine units to work with.

Indexing cam angles comparison (1/2)

In post [Rotary Indexer For Indexing Motion], we explained the meaning of indexing with the example of displacement and velocity diagram to give an idea how the indexing mechanism works. We also showed the location of input and output shafts of the rotary indexer. In this post, we're going to see how both shafts work and the meaning of indexing angle.

Geared motor and Turret plate connected to the rotary indexer

Basic equipment setup of indexing system is as shown in the picture. The input shaft of the rotary indexer is connected to the geared motor or other drive components e.g. pulley, gear, etc. In this example, we use a "hollow shaft" geared motor which allows indexer's input shaft to insert directly into the hollow gear shaft without using any shaft couplings. But they must be carefully align in order to avoid misalignment between shafts.

At the output side of the rotary indexer, the output shaft or flange can be mounted to a turret plate. There will be tools mounted on the turret plate at the same P.C.D. (pitch circle diameter). The tool at each station is usually work-piece holder. When the turret plate indexes, it will transfer the work-piece from one station to another station in order to complete all required processes at different stations.

Indexing motion from the rotary indexer doesn't require stopping of the motor since it has internal construction with cam and rollers that generate indexing motion at the output shaft while the input shaft runs continuously. As a machine designer, to select the right indexer for the application, the first thing to do is to select number of stations. Usually we will provide additional spared positions for future improvement i.e. if required number of stations is 6, we may select 8 stations instead.

Number of stations is usually "number of stops (S)" on the indexer. In the following picture, the indexer has number of stops = 4. In each turn of the input shaft (or geared motor's shaft), the output shaft (turret plate) moves 1/4 turn. Therefore, the displacement of the turret plate (h_m) in degree can be calculated using:

h_m = 360/S

where:
h_m = displacement of the turret plate (deg.)
S = number of stops

Number of stops (S), indexing angle (b_m) and displacement (h_m)

Hence, the displacement of the turret (h_m) is 360/4 = 90 deg. The displacement is also an important factor of the acceleration which we we explore more in later posts.

The indexing angle (b_m) is the total angle at the input shaft to rotate the output shaft (turret) to another station. If the rotary indexer has S=4 and b_m = 120 deg., it means that when the input shaft turns 120 deg., the turret will completely turn from one station to another with the displacement of 90 deg.

See how different indexing angles move in the next post.

Rotary Indexer for Indexing Motion

Rotary Indexer

Indexing is the process of intermittent motion where machine starts and stops at precise locations in specified intervals. Indexing motion is required in most machines for many applications since the stopping period (dwell period) of indexing allows other units in the machine do their jobs. If machine runs continuously without indexing, it may be much more difficult and complex for other units to follow the moving object compared with object that stops.

Indexing Motion

The easiest way to provide the intermittent motion is to start and stop the motor. Probably, a servo motor or a stepping motor can do that with precise intervals and locations. However, when required a high degree of rigidity, a positive motion mechanism to ensure no backlash, the cam-driven indexer is most suitable for the application. The indexer is available from many manufacturers which we can choose from product catalogs according to desired load, indexing interval, indexing profile, number of stops, etc.

Ideal Displacement and Velocity Diagram of a rotary indexer

The above chart shows 2 cycles of displacement and velocity of the rotary indexer. In each cycle, the rotary indexer rotates (indexing) then stops. The indexing time is the time of indexing and the dwell time is the time when the indexer has no movement (zero velocity). The following picture shows input and output shafts of the indexer.

The input shaft is normally connected to a geared drive (motor) that rotates at a constant speed. The construction inside the indexer (roller gear, cam, conjugate plate cams, etc.) makes the output shaft rotates and stops as shown in the earlier displacement diagram. However, in some applications, it may require longer dwell time for other machine units to do their jobs. For this case, the input shaft (motor) will rotate and stop and wait to extend the dwell time until other machine units have completed their processes then it rotates again. This is also called "cycle-on-demand" application.

Input and output shafts of a rotary indexer

To select the right rotary indexer for your application, there are several parameters to take into account such as number of stops, indexing angle, load (inertia), torque, etc. We will explore more details about those parameters. At the end we will make an excel calculation sheet to calculate values of necessary parameters for selection the right rotary indexer from the manufacturers.

Next: [Indexing Cam Angles Comparison (1/2)]

Reference:

• 3D CAD model (SANDEX right angle indexer: ED11)